Curves of Pursuit

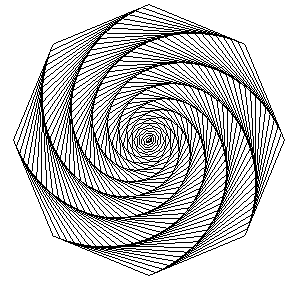
In preparation for my webinar, I came across something which I had seen before but never knew the name of: curve of pursuit. Suppose you have a triangle, and there was a mouse at each corner, staring directly at its neighbor on the clockwise corner, relative to itself. Suddenly, at the exact same time, the mice start chasing the one they are staring at. What kind of curve will be traced out by the mice? When will they meet? This curve, as it turns out, is a logarithmic spiral. What is interesting is that there are techniques for approximating these curves, and can be used for making all sorts of interesting designs. As fun as this is, it also struck me as an interesting demonstration of a dynamic system. The mouse is chasing a moving target, which is itself chasing a moving target. It’s an iterative system over differential time increments. The idea of limits and dynamic systems can be demonstrated using the drawing technique, since the finer the drawing (and hence, the more increments), the closer the approximation to the actual curve. I found a whole website devoted to different artsy math activities (https://www.artfulmaths.com/mathematical-art-lessons.html), and there is a whole page devoted to drawing curves of pursuit. She also talks about Celtic knots, symmetry, and origami. These activities seem like great ways to fill a Friday with something fun, yet genuine mathematical and educational.